
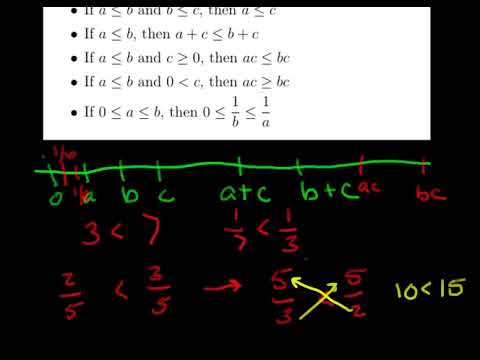
Similarly, dx + dx^2, with dx infinitesimal, was to be equal to dx due to the squared term being incommensurately smaller. The difference between infinitesimals lay in the assumption that because they were infinitely small, the difference between the sum of a (nonzero) real number and an infinitesimal and the real itself was negligible so the aforementioned quantities could be considered equal.
His Law of Continuity, for example, stated that familiar properties of the real numbers would still apply to infinitesimals. His rules for infinitesimals were heuristic, rather than formally proven, but were based on principles that intuitively ‘made sense’. Leibniz made early use of explicit infinitesimals in his formulation of calculus. This stands in contrast to the logic of Zeno of Elea, who reasoned, in his Paradoxes, that adding an infinite number of terms must yield infinity. Reasoning of this form requires implicit understanding that while an infinite number of terms are summed, they become infinitely small, yielding a finite sum. Archimedes reasoned, of the diagram, (below), that the shaded area must be ⅓ since it takes up ⅓ of each level of the fractal. To do this, he used a geometric argument, arranging pieces into congruent shapes that together formed a square.
Infinitesimals in the real number system series#
Human mathematical reasoning has been dealing with the concept of arbitrarily small numbers for millennia, and despite having been considered discredited with the advent of the limit, infinitesimals are still a useful way of looking at mathematics and the world.Īrchimedes summed an infinite series to calculate the area of general parabolic arches in his treatise The Quadrature of the Parabola.


 0 kommentar(er)
0 kommentar(er)
